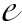Yesterday I wasted a ton of time playing against Shredder on my computer, and losing game after game… Finally I “dumbed the computer down” to a rating of 1977 just so that I would have a chance to win. And what do you know? I got a chance to play probably my nicest combination ever against the computer, a two-rook sacrifice (in effect). The sacrifice actually had three stages: an exchange sac, a bishop sac, and then a rook sac. The first two were accepted, but the third one was declined (for good reason).
The other cool thing is that this was, I believe, the first time I’ve played the Halloween Gambit against Shredder. That’s the crazy piece sacrifice that begins
1. e4 e5 2. Nf3 Nc6 3. Nc3 Nf6 4. g3?! Nxe4!?!?
There is also a “colors reversed” version of the Halloween Gambit that begins 1. e4 e5 2. Nf3 Nc6 3. Nc3 Nf6 4. Nxe5?!?! However, I consider that one to be not quite sound. The extra move 4. g3 actually works against White in two different ways. First, the pawn on g3 takes away what would be the best flight square for the knight. Second, by playing 4. g3 White has created weak light squares on the kingside that Black can exploit.
As always, it’s kind of hard to tell where White went wrong, but I got full compensation for the material and then some after
5. Nxe4 d5 6. Nc3 d4 7. Ne4 f5
In the Halloween Gambit you get to do exactly what your teachers said you shouldn’t — throw all of your pawns at the opponent.
8. Neg5 h6 9. Nh3 g5 10. Bb5 Qd5 11. Qe2 Bd7!
A key move. White can’t win a pawn with 12. Bxc6 Bxc6 13. Qxe5+ because of Black’s pressure on the long diagonal.
12. Bc4 Qd6 13. Nhg1 e4 14. d3 O-O-O 15. de fe 16. Nd2 Re8 17. Bf7 Re7 18. Bc4 …
This little bishop dance to f7 and back must be a mistake for White, as it gives Black a free tempo.
18. … Bg7 19. c3 e3 20. Kf1 ed
Winning back the sacrificed piece, with a huge positional advantage.
21. Qxd2 Rhe8 22. Kg2 Re1 23. Be2 …
 Position after 23. Be2. Black to move.
Position after 23. Be2. Black to move.
FEN: 2k1r3/pppb2b1/2nq3p/6p1/3p4/2P3P1/PP1QBPKP/R1B1r1NR b – – 0 23
This is one of those “Oh no, what have I done?” moments. In a tournament game I would have thought harder, but against the computer I just played 22. … Re1 because it seemed like such an obvious move. But after 23. Be2, my foolhardy rook is surrounded by a sea of White pieces! It almost looks like a misprint. What can I do?
Luck was on my side, as I have an unplanned but excellent move.
23. … R8xe2! 24. Nxe2 Qd5+! 25. f3 and now here comes sac number two: 25. … Bh3+!!
At first my thinking was just to draw White’s king away from g2 so that I can take his rook. But the trouble is that after 26. Kxh3 (which the computer played) now 26. … Rxh1? is not so good because after 27. Kg2! the rook is still trapped!
The solution? Another sacrifice!
26. … Qxf3!!
Forget about winning back the material — let’s checkmate Black’s king!
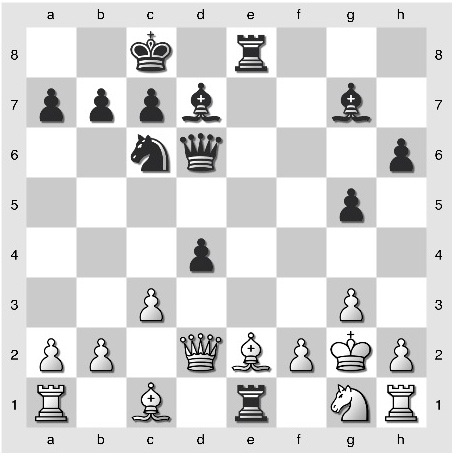
 Position after 26. … Qxf3. White to move.
Position after 26. … Qxf3. White to move.
FEN: 2k5/ppp3b1/2n4p/6p1/3p4/2P2qPK/PP1QN2P/R1B1r2R w – – 0 27
The proof of the pudding: If 27. Rxe1 then 27. … g4+ 28. Kh4 Bf6+ 29. Kh5 Qf5+ 30. Kxh6 Ne7!
This variation is worth another diagram:
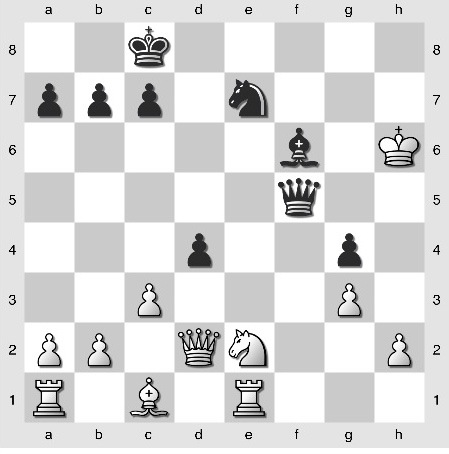 Position after 30. … Ne7 (analysis). White to move.
Position after 30. … Ne7 (analysis). White to move.
FEN: 2k5/ppp1n3/5b1K/5q2/3p2p1/2P3P1/PP1QN2P/R1B1R3 w – – 0 31
White is up two rooks but as Craig Mar likes to say, Black has a force advantage in the crucial sector of the board. A cute point is that if White plays 31. Nf4 to stop mate on g6, Black can play 31. … Ng8 mate instead. The knight, after being a bystander on c6 for the whole game since move 2, gets to strike the winning blow!
Of course the computer, even dumbed down to a rating of 1977, would not go for a line where it sees a forced mate. So in the second diagram above, it gave back the rook with 27. Nxd4 Qxh1. It’s kind of funny — here I thought, “I won a rook, so I’m winning the game.” It was a little bit of a shock to look at the position and realize that, because of my earlier sacrifices, I’m only back to even material!
Still, I was right. Black is winning, not because of the material but because White’s king is still exposed and White’s pieces are undeveloped. The game now continued 28. Qf2! Nxd4 29. cd and I had to make my last hard decision of the game.
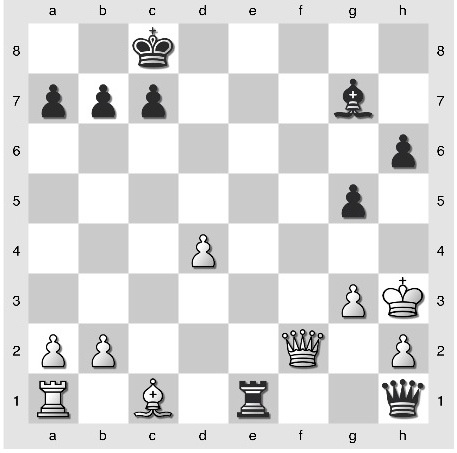 Position after 29. cd. Black to move.
Position after 29. cd. Black to move.
FEN: 2k5/ppp3b1/7p/6p1/3P4/6PK/PP3Q1P/R1B1r2q b – – 0 29
White has a cunning trap: 29. … Rxc1? 30. Rxc1 Qxc1 31. Qf7! would force Black to give back the bishop in order to avoid mate on e8. Darn computers! They never give up!
I looked for a long time at 29. … Qe4 and although I feel as if this must be winning, it still seemed to me as if 30. Qf7 posed some practical challenges.
Finally I calmed down and asked myself the Mike Splane question: How am I going to win this game? The answer is easy. I have an ironclad, unbreakable pin on the c1 bishop. If the queens came off the board, I would be winning. White’s queen is his only developed piece, so it’s completely logical for me to trade it off. I played 29. … Qf1+! 30. Qxf1 Rxf1 and the endgame was easily won after 31. d5 Bd4 32. Kg2 Re1 33. h4 g4, completing Black’s bind. I’ll skip the rest of the moves.
I know that it’s “just” a win against a computer, and a dumbed-down computer at that, but it sure was a satisfying game for me! From the mad-dog opening to the shower of sacrifices in the middlegame to the calm and methodical endgame, there wasn’t anything about it that I’d change. No, the only thing that I would change were the umpteen losses in a row that came before it…