As many of you know, I had a disappointing tournament in Reno last month, and I plan to write at least two or three posts trying to understand the reasons for my failure.
But I’d like to start with a “failure” that really wasn’t — it was an exquisite finishing combination by my opponent.
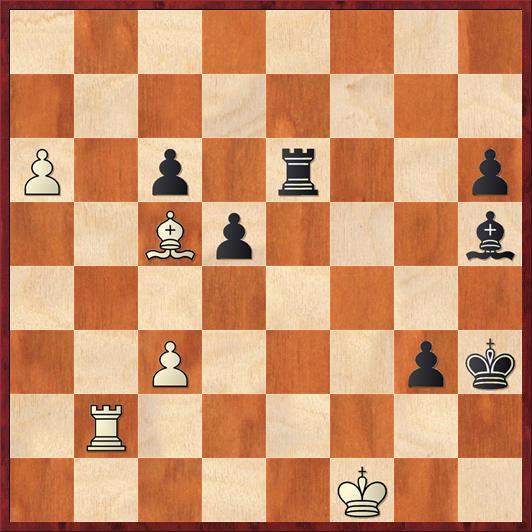
Position after 46. Rb2. Black to move.
FEN: 8/8/P1p1r2p/2Bp3b/8/2P3pk/1R6/5K2 b – – 0 46
I’m playing White here against Dale Haessel, a master from Canada. The opening was a King’s Gambit gone bad. In fact, both of my King’s Gambits in this tournament went bad in a similar fashion, and I will write another post about that. Anyway, I had been suffering for a very long time, but when we got to this point I actually thought that I had rescued my game, and in fact I wasn’t entirely sure how Black was going to stop my a-pawn.
As it turns out, this position doesn’t make a great puzzle position because there is more than one way for Black to win. During the game I thought that Black would have to play 46. … Bf3, because I assumed that he had to try to push his g-pawn. Then I analyzed 47. a7 and thought I was doing well. On 47. … g2? I play 48. Kf2, and there are no checkmates. Rybka, however, shows that I was wrong. Instead of 47. … g2, Black plays plays 47. … Re8! Now 48. Rb8? walks into mate in two, 48. … Bg2+ 49. Kg1 Re1 mate. So I have to play 48. Ra2, but then Black simply laughs at my naivete and plays 48. … h5! The point now is that whenever I queen, say 49. a8Q, Black will sacrifice his bishop: 49. … Bg2+! 50. Rxg2 (forced, to avoid mate) Rxa8. Black has three pawns for the bishop and White is in a really bad position to organize a defense. Rybka says the position is +3 pawns for Black.
However, Black is skirting disaster in the above line, and even though it works, Haessel found a combination that works even better:
46. … Bg6!
Of course I looked at this, but I didn’t see the point. As I mentioned in my last entry, I did a lot of one-move-deep “analysis” in this tournament, and this was one example.
47. Rd2 g2+! 48. Kf2 …
I saw that I couldn’t take the pawn because on 48. Rxg2 Bd3+ he will win my rook. (Black’s point is the bishop now checks on d3, where it is out of harm’s way, rather than on e2). Even so, I was still blindly certain that Black had nothing. After all, if 48. … Kh2 then I can play 49. Rd1. I am still stunned by the move that Haessel came up with next.
48. … Bh5!!
That’s right, he goes right back to the square he just came from! At first I thought, okay, he’s just taken the d1 square away from my rook, but I can just go back to b2 or a2. But gradually it dawned on my that I was completely lost! If 49. Ra2 Kh2! 50. Ra1 Re2 is mate! Equally beautiful is 49. a7 Kh2! 50. a8Q g1Q mate! If only I could play Kf3+ and then take on g1 with my bishop … but I can’t. There is absolutely no way around these twin checkmates. So I resigned.
This is the first appearance I have ever seen in a chess game — at least in one of my chess games — of a mathematical motif called conjugation. In math, conjugation arises when you “play a move” A, then another move B, and then you “take back” move A by playing the inverse of A. For example, if you are solving a Rubik’s cube you do this a lot. You want to move a certain square X to a certain spot with move B, but that move would mess up some other squares Y that you’ve already put in the right place. So you play move A to take arrangement Y “out of harm’s way,” then play move B to move X to its desired location, then you play the inverse of A to bring arrangement Y back to where it was initially.
Okay, if you’ve never solved Rubik’s cube, that is a bunch of gibberish. But we see exactly the same idea in Haessel’s combination. First he takes the bishop out of harm’s way with … Bg6. That enables him to play the move he really wants to play, which is … g2+. But then, after playing this move, he puts the bishop back on h5, because that is where he really wants it. On h5 it controls d1 and sets up the “twin checkmates,” Re2 mate and g1Q mate, that we saw above. Really, the bishop does three things at once, by controlling the key squares f3, e2, and d1.
Seeing this mathematical idea used in a chess combination for the first time thrilled me so much that I almost didn’t care that I lost the game.
(Almost.)
To conclude this post, I have a short announcement. Of course, the whole chess world knows that Fabiano Caruana and Magnus Carlsen are about to play their match in London for the world championship. For people in the Santa Cruz area, Gjon Feinstein and I will comment on three of the games in the match: games 2, 6, and (if it lasts that long) 11. We will get the live feed over the Internet but we will turn off the volume so that we can discuss the position without getting hints from the grandmaster commentary online. I’ve never tried live commentary before. It could be Fabi-lous fun, or it could be a fiasco! I don’t know which!
Anyway, if you would like to watch game 2, come to our World Championship Watch Party next Saturday, at Aptos Public Library, from 10 am to 12 noon!

Reno Lessons, Part One + Fabi-lous Fun!
by admin on November 3, 2018
As many of you know, I had a disappointing tournament in Reno last month, and I plan to write at least two or three posts trying to understand the reasons for my failure.
But I’d like to start with a “failure” that really wasn’t — it was an exquisite finishing combination by my opponent.
Position after 46. Rb2. Black to move.
FEN: 8/8/P1p1r2p/2Bp3b/8/2P3pk/1R6/5K2 b – – 0 46
I’m playing White here against Dale Haessel, a master from Canada. The opening was a King’s Gambit gone bad. In fact, both of my King’s Gambits in this tournament went bad in a similar fashion, and I will write another post about that. Anyway, I had been suffering for a very long time, but when we got to this point I actually thought that I had rescued my game, and in fact I wasn’t entirely sure how Black was going to stop my a-pawn.
As it turns out, this position doesn’t make a great puzzle position because there is more than one way for Black to win. During the game I thought that Black would have to play 46. … Bf3, because I assumed that he had to try to push his g-pawn. Then I analyzed 47. a7 and thought I was doing well. On 47. … g2? I play 48. Kf2, and there are no checkmates. Rybka, however, shows that I was wrong. Instead of 47. … g2, Black plays plays 47. … Re8! Now 48. Rb8? walks into mate in two, 48. … Bg2+ 49. Kg1 Re1 mate. So I have to play 48. Ra2, but then Black simply laughs at my naivete and plays 48. … h5! The point now is that whenever I queen, say 49. a8Q, Black will sacrifice his bishop: 49. … Bg2+! 50. Rxg2 (forced, to avoid mate) Rxa8. Black has three pawns for the bishop and White is in a really bad position to organize a defense. Rybka says the position is +3 pawns for Black.
However, Black is skirting disaster in the above line, and even though it works, Haessel found a combination that works even better:
46. … Bg6!
Of course I looked at this, but I didn’t see the point. As I mentioned in my last entry, I did a lot of one-move-deep “analysis” in this tournament, and this was one example.
47. Rd2 g2+! 48. Kf2 …
I saw that I couldn’t take the pawn because on 48. Rxg2 Bd3+ he will win my rook. (Black’s point is the bishop now checks on d3, where it is out of harm’s way, rather than on e2). Even so, I was still blindly certain that Black had nothing. After all, if 48. … Kh2 then I can play 49. Rd1. I am still stunned by the move that Haessel came up with next.
48. … Bh5!!
That’s right, he goes right back to the square he just came from! At first I thought, okay, he’s just taken the d1 square away from my rook, but I can just go back to b2 or a2. But gradually it dawned on my that I was completely lost! If 49. Ra2 Kh2! 50. Ra1 Re2 is mate! Equally beautiful is 49. a7 Kh2! 50. a8Q g1Q mate! If only I could play Kf3+ and then take on g1 with my bishop … but I can’t. There is absolutely no way around these twin checkmates. So I resigned.
This is the first appearance I have ever seen in a chess game — at least in one of my chess games — of a mathematical motif called conjugation. In math, conjugation arises when you “play a move” A, then another move B, and then you “take back” move A by playing the inverse of A. For example, if you are solving a Rubik’s cube you do this a lot. You want to move a certain square X to a certain spot with move B, but that move would mess up some other squares Y that you’ve already put in the right place. So you play move A to take arrangement Y “out of harm’s way,” then play move B to move X to its desired location, then you play the inverse of A to bring arrangement Y back to where it was initially.
Okay, if you’ve never solved Rubik’s cube, that is a bunch of gibberish. But we see exactly the same idea in Haessel’s combination. First he takes the bishop out of harm’s way with … Bg6. That enables him to play the move he really wants to play, which is … g2+. But then, after playing this move, he puts the bishop back on h5, because that is where he really wants it. On h5 it controls d1 and sets up the “twin checkmates,” Re2 mate and g1Q mate, that we saw above. Really, the bishop does three things at once, by controlling the key squares f3, e2, and d1.
Seeing this mathematical idea used in a chess combination for the first time thrilled me so much that I almost didn’t care that I lost the game.
(Almost.)
To conclude this post, I have a short announcement. Of course, the whole chess world knows that Fabiano Caruana and Magnus Carlsen are about to play their match in London for the world championship. For people in the Santa Cruz area, Gjon Feinstein and I will comment on three of the games in the match: games 2, 6, and (if it lasts that long) 11. We will get the live feed over the Internet but we will turn off the volume so that we can discuss the position without getting hints from the grandmaster commentary online. I’ve never tried live commentary before. It could be Fabi-lous fun, or it could be a fiasco! I don’t know which!
Anyway, if you would like to watch game 2, come to our World Championship Watch Party next Saturday, at Aptos Public Library, from 10 am to 12 noon!
Tagged as: conjugation, Dale Haessel, exquisite, Fabiano Caruana, Gjon Feinstein, King's Gambit, live commentary, Magnus Carlsen, mathematics, Rybka