dana blogs math: In Love With Geometry

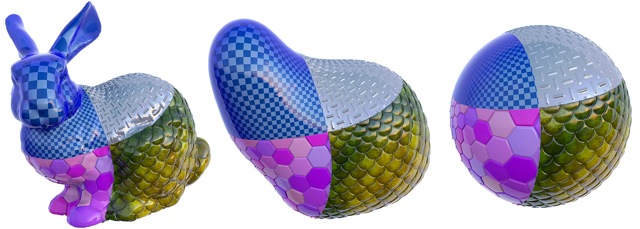
In this animation by Keenan Crane, a surface in the shape of a bunny flows to a sphere. In a "conformal" flow, like this one, all the surface patterns remain relatively undistorted. (Image courtesy of Keenan Crane.)

For generations, geometers have gotten used to not being able to see the objects that they prove theorems about. It’s a somewhat sad development in a subject that began in Euclid’s day with the splendidly visual concepts of points, lines, triangles, circles, conic sections and the like. But at the same time, it seemed like a necessary price for progress. Even in ancient Greece it became clear that pictures could fool you, and that abstract arguments (the “theorem-proof” approach of Euclid) were the best way to avoid making mistakes. Also, in the nineteenth and twentieth centuries, geometers moved to the study of much more complicated objects: surfaces and spaces of many dimensions that could never be fully visualized in our rather modestly endowed three-dimensional universe.

Keenan Crane. Photo by Katrin Schmid, courtesy of Mathematisches Forschungsinstitut Oberwolfach.

However, in the twenty-first century, mathematical computer scientists like Keenan Crane of Columbia University are giving a new life to the study of surfaces and curves we can actually see. Crane, who was selected as one of the 200 young participants in the Heidelberg Laureate Forum, works on computer visualization of objects from differential geometry. His work has placed him squarely at the intersection of both fields. “My background is really in computer science and computer graphics, but as I tried to solve these graphics problems I realized more and more that I was in love with geometry,” he says.

Looking at Crane’s pictures, it’s easy to see why. One of his current projects involves the deformation of surfaces by conformal maps. Suppose, for example, you want to morph a rabbit into a sphere, as shown in the animation above. Real-world objects will always have some surface coloration or texture on them, and aesthetically the most pleasing and most convincing “morphs” are the ones that don’t distort those patterns too much. That means that in any small patch of the surface, shapes are preserved. Eyes still look like eyes, and writing still remains legible even as the surface flows toward its new shape. Such shape-preserving transformations are called conformal.

Conformal mappings have a very distinguished pedigree in mathematics as well as in computer visualization. One of the landmark theorems of nineteenth-century mathematics, Riemann’s Uniformization Theorem, guarantees that any surface that is “topologically” a sphere—in other words, it has no boundaries and no handles—can be mapped conformally to a sphere. The proof, done in classical mathematical style, is very abstract and challenging even for a mathematics graduate student. When I was a graduate student, every one of my classmates boned up on this proof while preparing for their qualifying exams. It was a sort of rite of passage—the hardest thing we could be expected to know on the exam. (I’m not sure if anybody ever got asked about the Uniformization Theorem. It’s likely that the faculty knew about this ritual and therefore asked us about other things instead.)

Crane’s algorithm is essentially a visual illustration of Riemann’s theorem, and it is dead simple. No matter what shape of bunny you start with—it can even be a cow, or an octopus—his algorithm will find a way to deform it conformally to a sphere, and fast. The speed does not come from programming tricks. It comes from mathematical principles. Crane found a new way to describe surfaces, not in terms of the physical locations of the points on the surface, but by their curvature. The morphing process decreases the Willmore energy of the curvature. To put it more prosaically, it irons out any wrinkles as efficiently as possible.

Two different conformal flows that map a coffee cup to a doughnut. (Image courtesy of Keenan Crane.)

Although it’s cool to watch a cow or a bunny turn into a sphere, things get much more interesting when you apply conformal flow to surfaces with holes or handles in them. The second series of pictures illustrates a standard joke in mathematics: “A topologist can’t tell a coffee cup from a doughnut.” As the coffee cup deforms into a doughnut, the mathematical equations written on its surface remain highly legible, because of the conformal (shape-preserving) property of the flow.

Unlike spheres, doughnuts come in many different conformal types. Nineteenth-century mathematicians discovered a way of classifying them that is beautiful but highly abstract. (It’s called a complex structure, so that already gives you an idea…) It provides little visual intuition about what the roundest, most wrinkle-free doughnut in a given conformal class ought to look like. But by using his conformal flows, Crane is discovering a link between the complex structure and the appearance of the Willmore-energy-minimizing doughnut. “As the complex structure gets more twisted, the torus also gets more twisted,” he says.

You might say this is not surprising, perhaps even obvious. But it’s something that nineteenth-century mathematicians like Riemann could never prove, could never even guess at, because they couldn’t see the things they were working with. It’s another example of how the “Silicon Age” is making new mathematics possible.

A length-preserving flow untangles a complicated curve and reveals it to be homotopic (or topologically equivalent) to a figure eight. (Image courtesy of Keenan Crane.)

Even if you don’t understand their mathematical meaning, Crane’s visualizations are just plain pretty to look at. Take the third picture, for instance. It shows the idea of deforming a curve while preserving its turning number. You can think of carrying a compass with you as you drive around the curve, and asking how many times the needle goes around while you make a complete loop. If you’re driving around the complicated duck-shaped curve on the left, it’s hard to say. But for the figure-eight on the right, it’s easy. If you start in the middle, the needle goes around part way, then backs up to where it started. The net turning number is zero. Because the figure-eight curve came about from a smooth deformation of the duck curve, the duck curve must also have a turning number of zero.

Crane says that he doesn’t have any plans to meet or talk with specific people at the Heidelberg Laureate Forum; he is just looking forward to meeting “a lot of fascinating people.” Nevertheless, he would like to get a chance to meet Srinivasa Varadhan, one of the laureates attending the meeting. “I’ve been working on a project that uses the classic Varadhan formula to compute geodesic distance, and in fact I’m going to talk with some people at DreamWorks about it next month,” Crane says. [DreamWorks is the movie studio that brought you Kung Fu Panda.] “I’d like to tell Varadhan that I’m using his result to do something useful and practical. It’s fun to see an idea going all the way from math to something you can see on the movie screen.”

This blog post originates from the official blog of the 1st Heidelberg Laureate Forum (HLF) which takes place in Heidelberg, Germany, September 22 – 27, 2013. 40 Abel, Fields, and Turing Laureates will gather to meet a select group of 200 young researchers.

Dana Mackenzie is a member of the HLF blog team. Please find all his postings on the HLF blog.