The 2008 Berkeley International concluded yesterday, with Grandmaster Giorgi Kacheishvili of Georgia snagging the $1400 first prize by virtue of his last-round draw with Vinay Bhat. GM Zviad Izoria (also of Georgia)Â could have caught up with him if he had beaten Iryna Zenyuk, but the Bay Area WIM (Women’s International Master) finished off her excellent tournament by holding Izoria to a draw.
Also in the final round, David Pruess finally brought Irina Krush’s hot streak to an end, and Jesse Kraai finally won his first game of the tournament, against Lev Milman. Jesse was clearly off form, with one win, one loss and eight draws (two of them by half-point byes … so in games actually played, he had six draws).
In the norm watch, three people went home happy. As reported before, Rensch and Esserman earned IM norms. Also, Zenyuk’s last-round draw against Izoria gave her an IM norm to go with the WGM norm she had already earned.
Here are the final standings:
1. Kacheishvili, 7.5/10
2. Izoria, 7.0
3-5. Friedel, Krush, Rensch 6.0
6-7. Bhat, Pruess 5.5
8-12. Kraai, Sarkar, Sharavdorj, Esserman, Zenyuk 5.0
13-15. Milman, Naroditsky, Haessel 4.0
16. Evans 3.5
17. Kustar 3.0
I don’t have any games to show you because, well, my free one-week trial at the Internet Chess Club came to an end, and that is how I was obtaining the game scores. I’ve mentioned before that I am not a big fan of ICC, but if I’m going to do more live reporting (or almost-live) on chess events, I may have to change my mind and join up.
Finally, let me make a couple of addenda to my two most recent posts. After reading my discussion about the round nine pairings (see Round Eight: Photos, Norms and Space Heaters), John Donaldson asked me to post the following clarification.
Hi Dana,
I read your blog regularly so your discussion of why a 4.5 scorer was paired with a 3 pointer immediately caught my eye. Yes, to someone used to a 6 round event with 100 players, it would seem pretty odd to pair across a point and half but under the circumstances it was actually pretty predictable something like this would happen. Pairing the ninth round with only 15 of the original 19 players makes for a real challenge and the computer had to work from not only the top but also the bottom to make legal pairings. The situation will only be worse in the last round and exacerbated even more if there are additional byes and withdrawals. Had the event been 11 rounds there would have been the real possibility of having to possibly pair players against each other twice with no legal pairings possible for all.
John Donaldson
Let me just say that I hope no one perceived any criticism of the pairing method, implicit or explicit, in my post. My main intention was to report what the players themselves were discussing. John is quite correct that with such a long tournament and so few players (10 rounds, 17 players) some seemingly strange pairings were inevitable at the end. The tournament was more than halfway towards a round-robin.
I do not know anything about FIDE’s pairing rules, so I based my report on what the players told me. David Pruess told me that there is a rule that you can’t pair across more than two score groups. What this means is that if Rensch had 4.5, and there was a group of players with 4.0 and a group with 3.5, then it would be incorrect to pair him against someone with 3.0. It’s clear that Esserman also believed this to be the case, which is why he thought that a draw (which would have put him at 4.5) would ruin his chances of playing one of the two remaining foreign players (who were at 3.0).
I do not know whether a “two-score-group” rule actually exists in the FIDE rulebook, and even if it exists I do not know whether it would even apply in a tournament like this, where pairings outside of two score groups are inevitable at some point. In my opinion John Donaldson, who is an International Arbiter, is more likely to know the correct answer to these questions than the players are. That is why I wrote, “I am sure that John Donaldson would not have approved the pairing if he didn’t think it was legal.”
The moral is: We need more players next year!
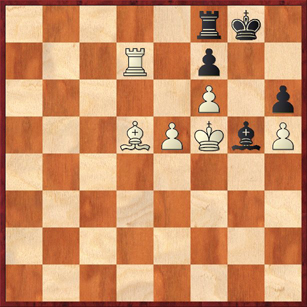
My second addendum relates purely to chess. Do you recall the following position from Izoria–Sharavdorj, discussed in my last entry?
Here Izoria (as White) ended the game with the pretty combination, 60. … Bh4 61. Rxf7! Rxf7 62. Bxf7+ Kxf7 63. e6 resigns.
I realized belatedly that White’s 62nd move probably deserves an exclamation point more than his 61st move. You might have wondered why Izoria didn’t simply play 62. Kg6, which would win back the whole rook, instead of giving up his bishop with 62. Bxf7+. The answer is that 62. Kg6?? would have thrown away the win because Black plays 62. … Kf8! (unpinning the rook) 63. Bxf7 (forced) Bxf6! The point is that if White recaptures the bishop either way, it’s a stalemate. If White doesn’t recapture, it’s now an easy draw.
So, paradoxically, it was better for White not even to have his bishop on the board! No bishop, no stalemates.
In the children’s chess club that I run, the rule that I have to explain the most often is castling. Second most often is the stalemate rule. Most beginners do not realize that a stalemate is a draw, and even after I explain it to them, some of them wonder why. The way I usually explain it is that the object of chess is to checkmate the opposing King, and if you can’t figure out how to checkmate him then you should not be rewarded with a victory. This is indeed the way that the question comes up in most kids’ games. One player will have two or three queens on the board but won’t be able to figure out how to checkmate, and will end up stalemating the opponent by accident.
However, there is a second reason for the stalemate rule, which I don’t usually tell the kids. In games between experienced players, stalemate-through-incompetence rarely happens. Nevertheless, the stalemate rule profoundly affects endgames, giving them more subtlety and richness. A lot of endgame paradoxes, like the one above, arise because of stalemate traps. I am a lover of paradox — it’s probably my downfall as a chess player, because I like to look for exceptions to the rule instead of following the rules. So the stalemate rule is one that I treasure, because it is a great source of exceptions. Just think how boring chess would be if a stalemate were a win. Every K+P versus K endgame would be a win for the side with the pawn, without exception. There would be no technique involved; brute material would always win. Bo-ring!
Okay, that’s all for now. I hope all of you who are reading this … all one or two of you … have a happy holiday! Drive safely! And don’t take any poisoned pawns!
